Frequently Asked Question
Background
What are you measuring?
We are measuring properties of space and time at the very smallest scales.
How small?
Very small! The "Planck length" (discussed more below) is $10^{-35}$ meters. One millimeter is 0.001 or $10^{-3}$ meters, so the Planck Length has a lot more zeroes after the decimal place. The Scale of the Universe 2 is a nice way to visualize length scales. Starting at $1$ meter, you can zoom "out" to the estimated size of the Universe ($10^{27}$ meters) and then zoom back "in" to fundamental particles. Notice that after the "smallest" particle, there is still a long way to zoom in before reaching the Planck length.
What new effect are you looking for?
The new idea we are testing states that positions (and time) are not precisely defined. When you measure the location of an object in two directions at the same time, the measurements have extra jitter.
We are seeking to measure a possible very slight random wandering of transverse position. This "holographic noise" could be caused by a new quantum uncertainty of space-time.
Why call it Holographic noise?
The theoretical ideas are similar to how ordinary holograms work. When you look through a hologram printed on a two-dimensional surface, a three-dimensional projection appears. Looking at this projection carefully, you see that it is a little fuzzy. This fuzziness is related to how small the pixels on the two-dimensional surface are. The smaller the two-dimensional pixels, the sharper the details are in the three-dimensional projection.
What is the basic strategy?
A Michelson Interferometer measures the x and y positions of the beam splitter (half silvered mirror) simultaneously. We monitor how much the beam splitter moves due to ordinary effects, such as vibrations from ground motion. We place two of these interferometers close to each other, so that the jitter from holographic noise from the two interferometers is coherent. This helps us measure the very small effect of holographic noise.
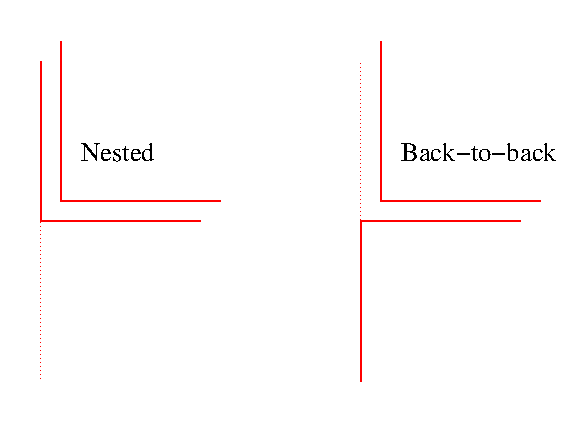
As shown in this figure, we will build the interferometers so they can operate in a "nested" or "back-to-back" configuration. The nested configuration maximizes the amount of coherence between the two interferometers. On one of the interferometers we can flip the direction of one of the arms while keeping everything else identical as much as possible. The holographic noise in this "back-to-back" configuration will not be coherent, and serves as an important test that we have correctly accounted for all sources of noise in the experiment.
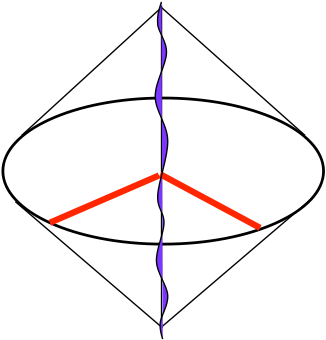
Can you explain your logo?
The two red lines represent the two arms of an interferometer, with the beam splitter located where the two arms meet. The vertical direction measures time. The point at the bottom of the logo is the time where a photon first hits the beam splitter. It propagates out both arms and reaches the two end mirror at the center of the logo. Continuing up, the reflected light recombines again at the beam splitter at the top of the logo. The path of the beam splitter is a wavy line to indicate the jitter in the position of the beam splitter.
What does holographic noise sound like?
Holographic noise is purely "white noise": it has the same amplitude at all frequencies. The sensitivity of our interferometers depends on frequency. At low frequencies we are 100% sensitive to the noise, and this decreases to zero at the frequency of a round-trip of light from the beam splitter to the end mirrors ($c/2L = 3.75$ MHz). At higher frequency there are overtones. Our ears are not sensitive to these frequencies, so we slow the noise down by a factor of 6000 to produce this audio file.
Where does the word "holometer" come from?
Holometer is short for "Holographic Interferometer". The twin correlated Michelson interferometers perform a holistic measurement of the quantum state of position of bodies over an extended volume of space-time. "Holometer" is also an archaic word. According to the Oxford English dictionary, in 1696, a Holometer was "A Mathematical Instrument for the easie measurement of any thing whatever."
Where are you building this experiment?
The Holometer is being built at Fermi National Accelerator Laboratory (FNAL) as Experiment E-990. We are reusing a portion of a tunnel that previously carried a polarized meson beam to MP9. This overview shows the location of the arms of the interferometers.

When did you start, and when will you be done?
In 2008 we had discussions with experients that use large interferometers to measure gravitional waves. The purpose of these discussions was to determine whether they had already detected this effect, or if they could be modified in a simple way to be more sensitive to it. Based on their advice, we started building prototype interferometers in May, 2009, which led to the design and construction of the full experiment. During the summer of 2013, we expect to be commissioning the detectors. Although it is possible that we will complete the measurement very quickly, we would not be at all surprised if it takes us a year or more to fully understand the apparatus so we can make a reliable measurement.
Theory
What is the Planck scale?
Physicist Max Planck proposed a set of units based on the values of fundamental physical constants. These constants are:
- Speed of light $c=3.0 \times 10^8$ meters/second;
- Gravitational constant $G=6.7 \times 10^{-11}$ meters$^3$/kilogram/second$^2$;
- Reduced Planck Constant $\hbar = h/2\pi = 1.1 \times 10^{-34}$ Joule seconds where $h$ is the Planck constant;
- Coulomb constant $1/(4\pi\epsilon_0) = 9.0 \times 10^9$ kilogram meter$^3$ /seconds$^2$/Coulomb$^2$ where $\epsilon_0$ is the permittivity of free space;
- Boltzmann constant $k_B = 1.4 \times 10^{-23}$ Joules/degree Kelvin.
Note that each of these constants is associated with a fundamental physical theory: special relativity ($c$), gravity ($G$), quantum mechanics ($\hbar$), electrostatics ($\epsilon_0$), and statistical mechanics ($k_B$).
We then combine these constants to define the Planck length, time, and mass:
- Planck Length $l_P = \sqrt{{\hbar}G/{c^3}} = 1.6 \times 10^{-35}$ meters
- Planck Mass $m_P = \sqrt{{\hbar}c/G} = 2.2 \times 10^{-8}$ kilograms
- Planck Time $t_P = l_P/c = \sqrt{{\hbar}G/c^5} = 5.4 \times 10^{-44}$ seconds.
These units seem to be limits on physically possible values. $c$ is the "speed limit" for how fast information propagates. Similarly, the $l_P$ could be a fundamental limit to measurements of position.
What does the Planck Scale mean for quantum mechanics and gravity?
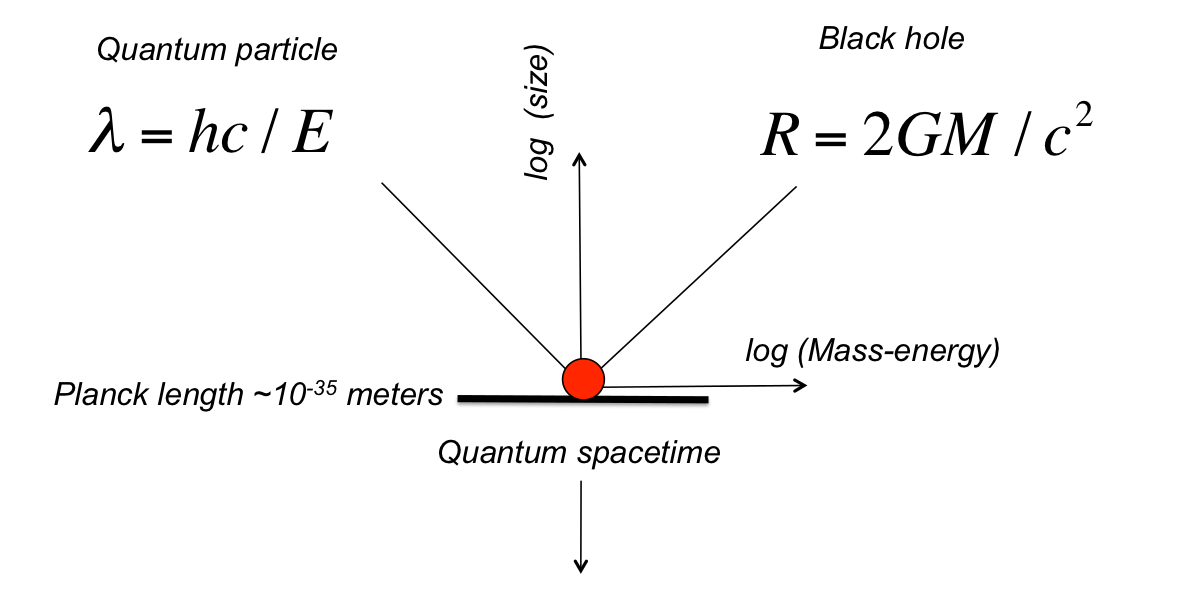
The graph below shows how the Planck scale relates to quantum mechanics and gravity. The vertical axis is length: wavelength for quantum mechanics, or radius of a black hole for gravity. The horizontal axis is energy: The energy of the light quanta for quantum mechanics, or the mass for gravity. Both axes are logarithmic. On the left, Einstein's photoelectric formula $E=hc/\lambda$ is a straight line with slope of negative 1. On the right, the radius of a black hole event horizon $R_{BH}=2GM_{BH}/c^2$ is a straight line of slope positive 1. At some size, the photoelectric $E$ will be equal to the $M_{BH}c^2$. Set this length $l = R_{BH} \sim \lambda$ and solve for $l=\sqrt{2hG/c^3}$ which is close to $l_P = \sqrt{hG/2{\pi}c^3}$. So, on the vertical axis of this plot, the realms of quantum mechanics and gravity "meet" at about the Planck scale. A black hole with a radius below the Planck length has less mass than a single quantum of that wavelength.
What is the holographic principle?
The amount of water a pitcher can hold is simply its volume. We ordinarily think that the capacity of a container is related to its volume. However, the holographic principle states that the capacity of a container is related to its surface area rather than its volume.
This is similar to how ordinary holograms work. We look through a two-dimensional plate to view a three-dimensional scene.
How does holographic noise relate to the holographic principle?
We use the holographic principle to calculate the strength of holographic noise. To do this, we set two quantities equal to each other: the degrees of freedom of position wave functions and the entropy of a black hole event horizon.
If we do not detect holographic noise, it means that the number of position states exceeds the holographic bound, in contradiction to the holographic principle.
What does this have to do with Heisenberg's Uncertainty Relationship?
The uncertainty relationship describes what happens when measuring two properties simultaneously. For example, if we measure the position on a direction axis ($x$) and momentum along that same direction ($p_x$), there is a limit on how well we can measure both of these quantities: ${\Delta}x {\Delta}p_x \ge {\hbar}/2$.
For holographic noise, the two properties are positions measured in different directions. So, if we measure the position on one direction axis ($x$) and position along a perpendicular axis ($y$), the limit on how well we can measure both of these quantities is ${\Delta}x {\Delta}y \ge l^2_P/2$.
How small is the effect you are looking for?
If it exists at all, we have a pretty good idea of its amplitude, fixed by the Planck scale. This scale combines the Planck constant of quantum mechanics with $G$, the constant of gravity.
In our apparatus, the amplitude of the motion is predicted to be about $\sqrt{{l_P}{L}}$, where L is 40 meters, at frequencies of a few megahertz, corresponding to about ten attometers ($10 \times 10^{-18}$ meters) of motion in a third of a microsecond. The velocity is about a millimeter a year, or about ten times slower than continental drift.
What does it mean to say "a particle confined to a Planck volume automatically collapses to a black hole?"
The radius of a black hole is given by the Schwarzschild radius. If the black hole has a mass $M$ its event horizon has a radius $r_s = 2GM/c^2$. For an object with the mass of the Sun, this radius is about 3 kilometers.
If we work out this radius for a particle with the Planck Mass, we get $r = 2GM_P/c^2 = 2G\sqrt{{\hbar}c/G}/c^2 = 2\sqrt{{\hbar}G/c^3} = 2l_p$. So, if you compress a something with one Planck mass, the event horizon (Schwarzschild radius) is 2 Planck lengths.
However, it is not clear that these equations are valid at the Planck scale. The position of quantum particles is defined by wave functions, and in quantum mechanics their position is always inherently fuzzy. There is no instant when they are perfectly localized. In quantum mechanics, a more localized particle has more energy. But this energy eventually becomes enough to distort the curvature of space itself by gravity, so the properties of space automatically enter the quantum realm. This is what we don't have a complete theory for -- quanta of space and time.
How is holographic noise consistent with stringent tests of Lorentz violation?
None of the stringent tests of Lorentz violation performed would have detected the particularly subtle effects of holographic noise. It is simply too small to have a detectable effect on laboratory experiments with atoms or molecules. There is no effect at all on measurements of particles propagating from far away, because they only measure one direction of space.
Holographic noise arises from a quantum indeterminacy of position compared in two directions, but the physics defines no preferred direction, position or velocity, except that defined by a particular measurement, as is usual in quantum mechanics.
How is holographic noise different from space-time foam?
John Wheeler's vision of quantum space-time was a roiling foam of virtual black holes. It was based on extrapolation of quantum field theory to the Planck scale. The holographic view is that space-time is not quantized like other fields, but emerges from a quantum system with fewer degrees of freedom than field theory. If this is right, foam is not the right way to visualize the smallest scales.
What do you mean by emergent space-time?
In general, we say that a property is "emergent" when it is obvious at large scales, even though it does not exist on smaller scale. Here is an example. The color of a wall is a large scale property. The color of the entire wall is the same as the color of individual drops of paint. However, when we get to a very small scale, the idea of "color" does not work. The color we see depends on how the fundamental particles (electrons, neutrons, and protons) are arranged. But each of these fundamental particles does not have a color.
Similarly, we can say that space-time itself is "emergent" from a fundamentally quantum system. On large scales is looks like what we are used to. However, on small scales the concepts of position do not exist. This happens at about the level of the Planck length. The concept of time does not exist for intervals shorter than the Planck time. This fundamental quantum system "looks like" classical spacetime in a macroscopic limit.
What is the classical limit of the holographic noise model?
On larger scales, the system becomes more classical: the fractional wobble in angle gets smaller. So, for all observations to date, the effects of this noise are negligible. On the other hand, the amplitude of the motion actually increases as the square root of the size. That is why the effect is detectable at all in our experiment.
In the limit of small Planck length or Newton's constant, the effect also diminishes.
Why is there noise in a time series?
In our experiment, we are continously sampling each interferometer, measuring the difference in arm lengths. We make measurements at a rate of 100 MHz, or once every 10 nano seconds.
As usual in quantum mechanics, the entire wavefunction is never observed directly in a physical measurement. The uncertainty in positions due to holographic noise translates into noise in this time series of measurements. The photons in the interferometer for the 10 nano seconds measure the position in each direction by physical interactions with matter. The ongoing collapse (or branching) of the wave function with time creates a new kind of noise in nonlocal, transverse position differences.
Why do we expect the nested interferometers to have correlated holographic noise?
The effect is consistent with existing experiments if the position wave function represents a deviation from a classical trajectory that is shared coherently and non-locally. That is, all bodies in a small region (where "small" is defined relative to the separation) share approximately the same deviation in a measurement that collapses the wave function. This interpretation is consistent with the idea of an emergent space-time; the wavefunction describes a spatial relationship between world lines that depends only their positions, and not on any other properties of bodies being measured. Because the spectrum of the uncertainty produces smaller amplitude displacements on small scales, it is also consistent with current experiments.
Why exactly do we expect the signal to vanish in the back-to-back configuration?
In this configuration, the beamsplitter position is referenced to two end mirrors in opposite directions. The measurements refer to regions of time and space that have nothing to do with other, so the correlation should vanish. Another point is that the beamsplitter surfaces are at right angles to each other in this configuration. On average, reflections from these surfaces measure exactly orthogonal components of motion, which should be independent.
How does the theory of position tested by the Holometer differ from other interpretations of noncommutative geometry?
The assumption is made that position operators described by the noncommutative geometry apply to positions of massive bodies. Most treatments apply the tools of quantum field theory, which amounts to a different theory of position. Also, the position operator is interpreted physically in terms of interactions with radiation in each direction. In effect the coordinate system of position can be thought of as defined by plane waves of radiation. The commutator of position is between these directionally-defined position operators.
How does holometry relate to string theory?
Nobody has yet developed this connection into a mature theory. There may be connections with one form called matrix theory, but here again, the theory of position of massive macroscopic bodies is not mature.
Certain systems in string theory display holographic properties. The most famous is Anti-deSitter space, where a field theory on the boundary is shown to correspond to gravity in the "bulk". However, in all of these systems, gravity and space curvature are critical to understanding the holographic properties, so they do not illuminate the flat-space degrees of freedom we probe in the laboratory.
Where does holographic noise tie in to other holographic theories? Where does the part about there only being two dimensions come in again?
Holographic theories come from ideas about gravity, and holographic noise is about unaccelerated systems in a flat space. So the connection is not obvious. But the information content of positions in the space is actually holographic. To see this, assume that position in a 3-sphere of radius $R$ is effectively "pixelated" with uncertainty of order $l_P$ in the radial direction, and uncertainty from holographic noise in the transverse (tangential) directions. The number of independent positions in the three diemensional volume then scales like $(R/l_p)(R/{\Delta}x_i)(R/{\Delta}x_j) \sim (R/l_P)^2$ which increases as a surface area rather than as a volume.
Experiment
How do you make this measurement?
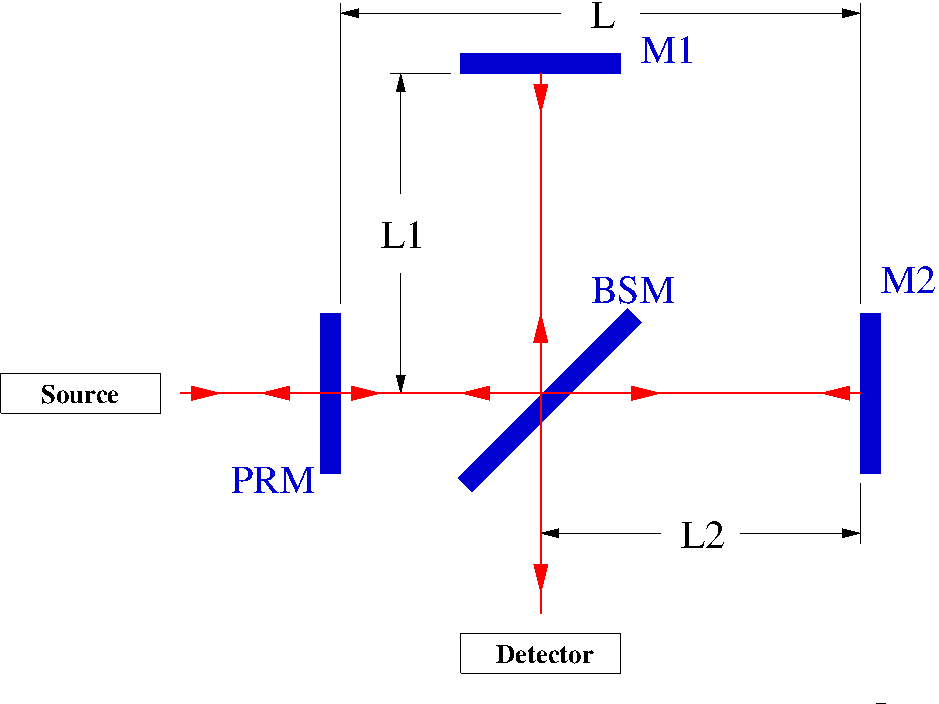
We operate a power recycled Michelson Interferometer and monitor the $(x,y)$ position of the beam splitter as a function of time.
The source sends monchromatic photons into the interferometer from the left. Each photon strikes the power recycling mirror (PRM). The probability that the photon will reflect off the PRM depends on the reflectivities of the mirrors and on the total length of the interferometer ($L$ in the diagram). If $L$ is exactly an integral number of wavelengths of the photon, then the photon is most likely to be absorbed into the cavity. We use a laser which allows us to control the wavelength of light it produces. We monitor the number of photons that do not get absorbed into the interferometer and set the wavelength of light to maximize the number of photons absorbed into the interferometer.
When a photon meets the beam splitter mirror (BSM) its quantum state changes to a combination of two states, one propagating up and the other propagating to the right. After reflection from the two end mirrors (M1 and M2) these states recombine and the direction of propagation depends on the difference in arm lengths $L1-L2$. We monitor light at the detector, and tune the path length difference ($L1-L2$) to hold the number of photons reaching the detector to a set level. The remaining photons propagate to the left, and then are reflected by the PRM to repeat the process.
How can you detect the holographic noise when there are so many other sources of noise from the environment?
The mirrors are not perfectly stationary. One source of noise is mechanical vibrations from seismic motion and other activity in the area, such as doors opening and closing. We measure the signal at the detector, and then move the positions of M1 and M2 to lock the signal at a set level. We keep track of how much the signal varies from the set level, and this measures the total amount of noise in the system. We can "see" when someone opens and closes a door. These sources of mechanical noise are large at low frequencies, and fall off at higher frequencies where we will search for holographic noise. Even if mechanical noise affects two interferometers coherently, we will distinguish this from holographic noise because of the dependence on frequency: mechanical noise decreases as 1/frequency while holographic noise is constant with frequency.
Other sources of noise are due to the electronics we use to record signals from the detectors, and statistical noise due the limited (though quite large) number of photons in the interferometers. The two interferometers will use independent light sources and electronics, so these noise sources will not be coherent. A final check that we have isolated the two interferometers from each other completely is the comparison of the correlated holographic noise in the "nested" and "back-to-back" configurations.
The experiment is designed with just this issue in mind. The thinking is that if we have two beamsplitters in separate sealed tubes, the light hitting them will be random but uncorrelated. A correlated part will come from acoustic shaking, but at very high frequency this part should be very small, and also not matched in length scale, because those waves travel much slower than light. The holographic cross-correlation from the interfered light has a very specific signature in frequency.
Would other noise from the environment be correlated as well?
That is a concern. Electromagnetic radiation, for example, could cause correlations in the two interferometers. However, these will be at specific frequencies, rather than the "white noise" of holographic noise.
The combination of three factors will help convince us that we have detected holographic noise:
- the strength of the correlated signal, compared to theoretical predictions
- the spectral shape (white noise modified by the frequency-dependent sensitivity of our interferometers
- the modulation of the correlation between the nested and back-to-back configurations
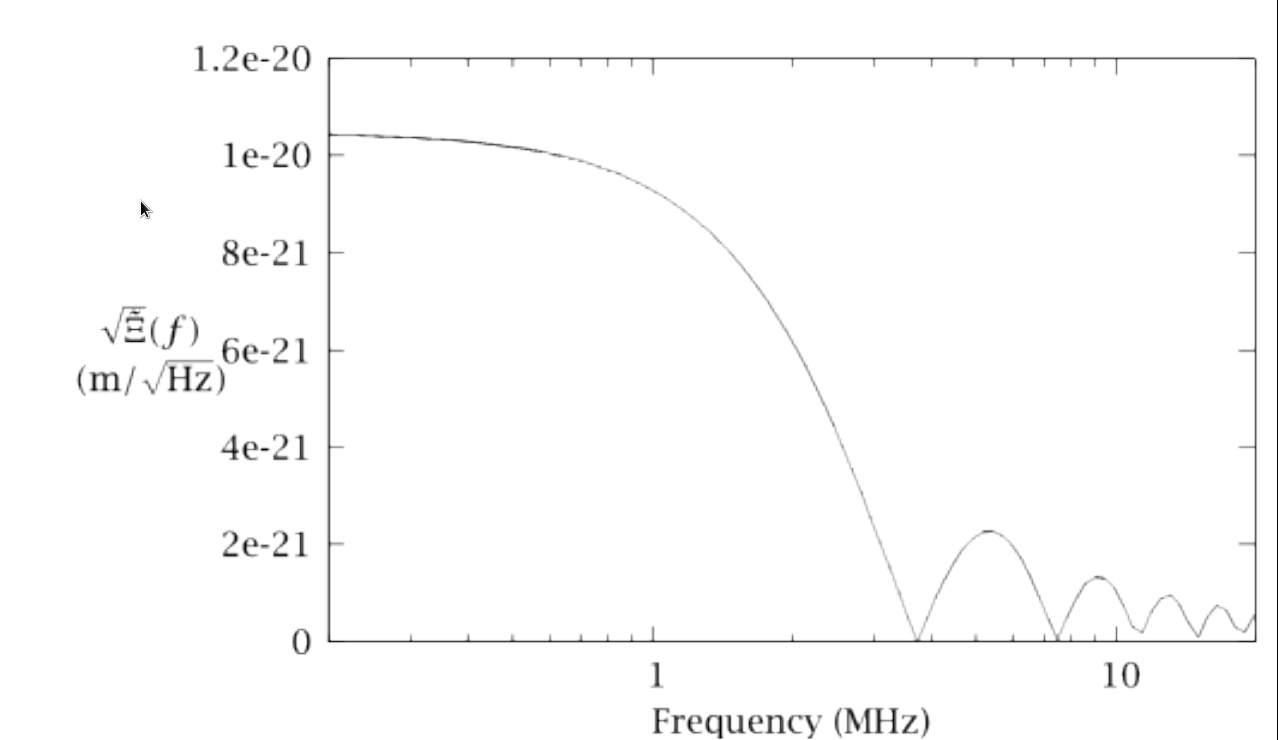
Why 3.75 MHz?
Holographic noise is constant at all frequencies. The interferometers, however, are not 100% efficient at all frequencies. They have no sensitivity at the frequency of light making the round trip from beam splitter to end mirror and back: $c/2L_1 = 3.75$ MHz for our arm length of $L_1 = 40$ meters. This plot shows the amplitude of the holographic noise multiplied by this efficiency.
What kind of laser do you use?
We use a solid state laser that produces infrared light with a wavelength of 1064 nano meters. It uses a crystal of Nd:YAG (neodymium-doped yttrium aluminium garnet; $\mathrm{Nd\!:\!Y_3Al_5O_{12}}$) and runs in continuous wave (rather than pulsed) mode with about 2 Watts of power.
Why don't you use a $\mathrm{CO_2}$ laser?
Although $\mathrm{CO_2}$ lasers are available with higher power, the main advantage of the solid state laser we us is that the frequency is more stable. (It has a smaller linewidth, or ${\Delta}\nu$.) Also, the wavelenth of light from $\mathrm{CO_2}$ lasers is about ten times longer, reducing the sensitivity measuring accurate positions. Finally, it is much easier to purchase optics that work at the 1064 nano meter wavelength.
How good are the mirrors that you use?
The optics are ground to the correct shape to within a fraction of a micron. The coatings make them very reflective to 1064 nano meter light. Typically, less than 30 photons per million are not reflected from the mirrors we use.
How long will it take to measure holographic noise?
In our proposal we calculate in equation 15 the exposure time as $$ t_{obs} \gt \left(\frac{h}{P_{BS}}\right)^2\left(\frac{\lambda_{opt}}{l_P}\right)^2\left(\frac{c^3}{32{\pi}^4L^3}\right)$$ where $P_{BS}$, the power on the beam splitter, is 2000 watts, $L$, the length of the interferometer arms, is 40 meters, and $\lambda_{opt}$ is the wavelength of the light we use, 1064 nano meters. At least 1/2 hour is needed to detect a significant (3 sigma) amount of holographic noise. Imperfections in the interferometers due to misalignment and optical distortions tend to increase this time.
What other large interferometers are there?
There are several large interferometers designed to detect gravity waves.
Do they see holographic noise?
LIGO's correlation study avoided nearly co-located interferometers, which are needed to see the holographic effect. They had to avoid them because at low frequencies studied for gravitational waves, all sorts of correlations would have been introduced by the acoustic environment. The LIGO interferometers are not configured to have power recycling to have a large $P_{BS}$, so they are not sensitive to holographic noise.
The published GEO600 noise is within a factor of two of the expected holographic noise level. There are uncertainties of the order of a factor of $\pi$ in the exact prediction for holographic noise, so GEO600 will have to improve considerably to rule the idea out conclusively.
Does holographic noise affect measurement of star positions, where the light has propagated cosmological distances?
Holographic noise does not affect photon propagation, only matter position. The effect over a cosmological distance is a random motion of the apparent position of a star of about 0.1 millimeters, over about ten billion years. This is not detectable.
What about the INTEGRAL results that showed no difference in polarization of gamma rays at different energies? Does this rule out the theory of holographic noise?
The quantum-geometrical noise in position we are looking for has no effect on propagation of particles moving in just one direction, such as particles from distant cosmic sources. It can only be detected by comparing position in two different directions, which is why the Holometer needs two arms at right angles.
Interpretation
If the Holographic Principle were accepted how would the fundamental concepts of physics be affected?
We would have a clue to the quantum origin of space-time -- how it grows up out of some new physics on very small scales. There are many ideas for how this might work, but none of them currently is tested by an experiment. Therefore, theories would have to become much more practical and concrete.
Do nearby surfaces really have information encoded on them?
The idea of encoding here is mathematical. It refers to spatial relationships in a representation of reality. There's nothing really "there" at the surface of a black hole; it is just an imaginary surface in empty space.
If space is digital, what happens as we move from one point to another? If we can't be in between two points, do we teleport over very small distances?
In some sense, things can be in two places at once. This is actually part of the standard lore of quantum mechanics. We are extending that idea to the points themselves. The closer two points are to each other, the less different they are.
This kind of ambiguity is familiar both in quantum mechanics and information theory. Claude Shannon proved a famous "sampling theorem" that if you have a function that does not contain any frequencies above a certain cutoff, it can be completely specified by a finite set of numbers, two for every wave period. It is a continuous function but the information is not independent for nearby points.
How can you seriously claim that we're all just living in a simulation? How can you seriously claim that the third dimension doesn't exist? Have you gone off the deep end? Don't you have anything better to do?
Simply put, the Holometer is testing none of these claims. We can address some of the misconceptions about the Holometer by clarifying several misleading terms.
First, when physicists say the universe is "really" two-dimensional, they don't mean the third dimension doesn't exist. Rather they mean it's an emergent rather than fundamental property of spacetime. If you zoom in far enough, a solid doesn't look very solid at all, but this doesn't make "solid" any less real or valid a category for describing our day-to-day experience. Similarly, the claim is that at some scale, spacetime can be described mathematically using two dimensions instead of three, and as you approach the scale of everyday life, it begins to look increasingly three-dimensional.
Second, the term "holographic" unfortunately calls to mind words like "illusion" and "simulation" which really have nothing to do with the Holometer or any aspect of the Holographic Principle. The notion that our familiar three-dimensional universe is somehow encoded in two dimensions at the most fundamental level does not imply that there is anybody or anything "outside" the two-dimensional representation, "projecting" the illusion or "running" the simulation. The Holometer may or may not find evidence of holographic noise. But it's a pretty safe bet that it will not call into question the reality or meaning of your life.




